The Radial Distribution Function, R.D.F. , g(r), also called pair distribution function or pair correlation function, is an important structural characteristic, therefore computed by I.S.A.A.C.S..
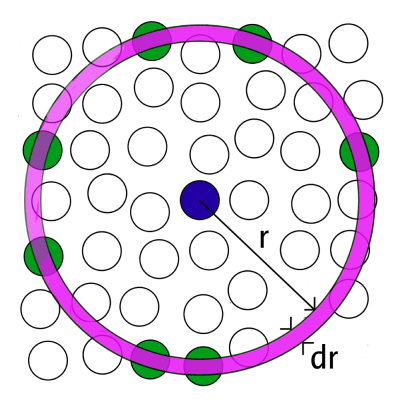
Considering a homogeneous distribution of the atoms/molecules in space, the g(r) represents the probability to find an atom in a shell dr at the distance r of another atom chosen as a reference point [Fig. 1].
By dividing the physical space/model volume into shells dr [Fig. 1] it is possible to compute the number of atoms dn(r) at a distance between r and r + dr from a given atom:
where N represents the total number of atoms, V the model volume and where g(r) is the radial distribution function.
In this notation the volume of the shell of thickness dr is approximated ![]() Vshell =
Vshell =  π(r + dr)3 -
π(r + dr)3 -  πr3
πr3 ![]() 4π r2 dr
4π r2 dr![]() .
.
When more than one chemical species are present the so-called partial radial distribution functions gαβ(r) may be computed :
where cα represents the concentration of atomic species α.
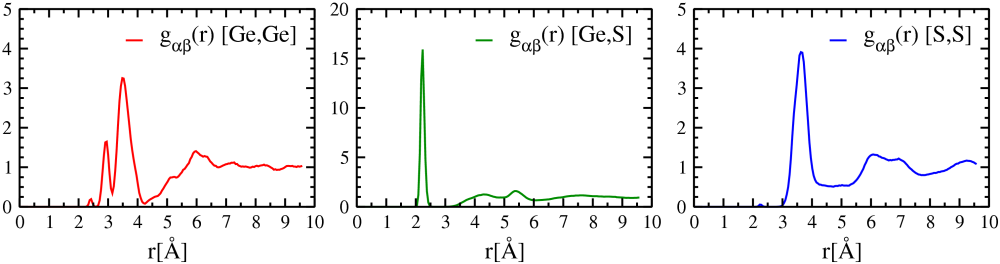
These functions give the density probability for an atom of the α species to have a neighbor of the β species at a given distance r. The example features GeS2glass.
Figure [Fig 2] shows the partial radial distribution functions for GeS2glass at 300 K. The total RDF of a system is a weighterd sum of the respective partial RDFs, with the weights depend on the relative concentration and x-ray/neutron scattering amplitudes of the chemical species involved.
I.S.A.A.C.S. gives access to the partial distribution functions gαβ(r) as well as to the partial reduced distribution functions Gαβ(r) defined by:
Two methods are available to compute the radial distribution functions:
- The standard real space calculation typical to analyze 3-dimensional models
- The experiment-like calculation using the Fourier transform of the structure factor obtained using the Debye equation

|
|
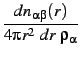 with ρα =
with ρα =